diff options
Diffstat (limited to '')
| -rw-r--r-- | 08.-Passive-Radar.md | 30 |
1 files changed, 16 insertions, 14 deletions
diff --git a/08.-Passive-Radar.md b/08.-Passive-Radar.md index 7a574df..3396556 100644 --- a/08.-Passive-Radar.md +++ b/08.-Passive-Radar.md @@ -81,30 +81,32 @@ If you are using wideband illuminators such as HDTV or 4/5G signals, you want to If you are using DAB (or other more narrow illuminators), note that this only has a bandwidth of about 1.5 MHz. Therefore you will obtain better results if you adjust your bandwidth to match as you don't want to receive the noise floor along the sides of the illuminator. From a base of any pr_ preconfiguration, under the DAQ reconfiguration settings, change the sample rate to 1.4 MHz. And then hit the "Reconfigure & Restart DAQ chain" button. This may take 1-2 minutes before the software has restarted. -# Range Doppler Units -The range-doppler graph displays bistatic range and bistance speed. The axes are currently described in CELLS. In order to convert cells to meters or m/s use the following formulas: +# Range-Doppler Units +The range-doppler graph displays bistatic range and bistatic speed. The axes are currently described in CELLS. In order to convert cells to meters or m/s use the following formulas: -Cell To Meters = cell * c/fs +$Bistatic Range (meters) = cell * c/fs$ -Where c is the speed of light, and fs is the sample rate. +Where $c$ is the speed of light, and $fs$ is the sample rate (aka bandwidth), and $cell$ is the x-axis cell. The sample rate by default is set to 2.4 MHz. If you have an illumination signal that is smaller, you should set your sample rate to the closest possible bandwidth that matches that illumination signal. -So for example if we see an object at cell 50, and we have a sample rate of 2.4 MHz (assuming an illumination signal at least that large too) We can go 50 * 299792458 / 2400000 = 15,614m = 15.6 km (bistatic distance) +So for example if we see an object at cell 50 on the x-axis, and we have a sample rate of 2.4 MHz we can calculate $Bistatic Range (meters) = 50 * 299792458 / 2400000 = 15,614m = 15.6 km$ -For doppler: +For converting doppler cells to Hertz the formula is as follows: -Cell to Hertz = cell * fs / (2*N) +$Bistatic Frequency (Hz) = cell * fs / (2*N)$ -Where fs is the sampling frequency and N is the sample size of the coherent processing interval. +Where $fs$ is the sampling frequency as before, $N$ is the sample size of the coherent processing interval (CPI), and $cell$ is the y-axis cell on the graph. -Example cell 100, sampling rate 2.4 MHz and N = 2^22 +In the passive radar software we provide three configuration files `pr_2ch_2pow20`, `pr_2ch_2pow21`, `pr_2ch_2pow22`. In these files the difference is in the CPI value, which is set to $2^{20}$, $2^{21}$ and $2^{22}$ respectively. -Doppler Frequency = 500 * 2400000 / (2*2^22) = 143 Hz +Example cell 100, sampling rate 2.4 MHz and $N = 2^{22}$ -To get to m/s we simply multiple the doppler frequency with the wavelength of the illuminator, and multiple by -1. (Positive Doppler decreases the range between you and the target so it has negative speed, it is approaching) +$Bistatic Frequency (Hz) = 500 * 2400000 / (2*2^{22}) = 143 Hz$ + +Then to get to speed in m/s we simply multiple the doppler frequency with the wavelength of the illuminator, and multiply by -1. (Positive Doppler decreases the range between you and the target so it has negative speed, it is approaching) So if we were using 560 MHz as our illuminator: -Bistatic Doppler in m/s = c / f * -doppler_freq = 299792458 / 560000000 * -143 = 76.5m/s = 275 km/h +$Bistatic Speed (m/s) = c / f * -doppler_freq = 299792458 / 560000000 * -143 = 76.5m/s = 275 km/h$ # Bistatic Range The graph provided is a bi-static range doppler graph. Bi-static means that the measurement consists of a transmitter and receiver separated by some distance. This can get complicated, as instead of getting a simple range distance value from the receiver, we end up with an ‘constant range ellipse’ of possible range solutions that depend on some calculations based on the transmitter and receiver positions. @@ -113,9 +115,9 @@ In the future we aim to have software enhancements that make understanding and v 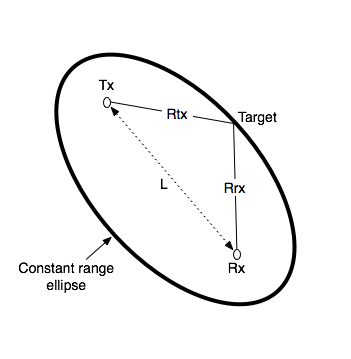 -The bistatic range displayed on the KrakenSDR range-doppler graph is described by the formula Rtx + Rrx - L +The bistatic range displayed on the KrakenSDR range-doppler graph is described by the formula $Bistatic range (meters) = Rtx + Rrx - L$. So you can see that a single reading on the range-doppler graph describes an ellipse of possible locations. # Range Resolution -Range resolution depends on the sampling bandwidth, which for the KrakenSDR and RTL-SDR tuners inside is 2.4 MHz. Therefore we achieve c / fs = 299 792 458 / 2400000 = ~125m resolution per range cell on the graph (assuming the illuminating signal is at least 2.4 MHz as well). +Range resolution depends on the sampling bandwidth, which for the KrakenSDR and RTL-SDR tuners inside is 2.4 MHz. Therefore we achieve $c / fs = 299 792 458 / 2400000 = ~125m$ resolution per range cell on the graph (assuming the illuminating signal is at least 2.4 MHz as well). This means that we can differentiate between two different objects that are 125m apart.
\ No newline at end of file |
